
Radii of the circle with center $O$ and radius $|OP|$. We also know that $|PO| = |QO|$ because both are We have $|PO| = |PQ|$ since both are radii of the circle with center $P$Īnd radius $|OP|$. If we draw a circle of radius $|OP|$ centered at $P$ it will meet theĬircle $C$ in two points, labelled $Q$ and $U$ in the picture below: Segment $OP$ and successively build equilateral triangles from here. In order to construct our hexagon, we can start with $60$ degrees, the two base angles must also measure $60$ degrees making itĪn equilateral triangle. On the circle $C$ is an isosceles triangle since all radii of $C$ have the same length. A triangle with vertex $O$ and its other two vertices We can find it when all the sides are known and add them together.Since a regular hexagon divides the circle into six equal parts and there are $360$ degrees in the circle, each side of the regular hexagon should span a chord of There is no definite formula to find the perimeter of an irregular hexagon. Here we will use Heron’s formula to find the area of each triangle.Īrea (A) = √s(s – a)( s– b)(s – c), here s = ½ (a + b + c) a, b, and c are the 3 sides of any triangle Let us solve an example to understand the concept better.Īrea of the hexagon ABCDEF = Area of ΔABC + Area of ΔACF+ Area of ΔFCE + Area of ΔECD There is no definite formula to find the area of an irregular hexagon. Let us see how to calculate the area and perimeter of an irregular hexagon. We can find the area and perimeter of a regular hexagon very easily.

In contrast, an irregular hexagon does not satisfy the above features.

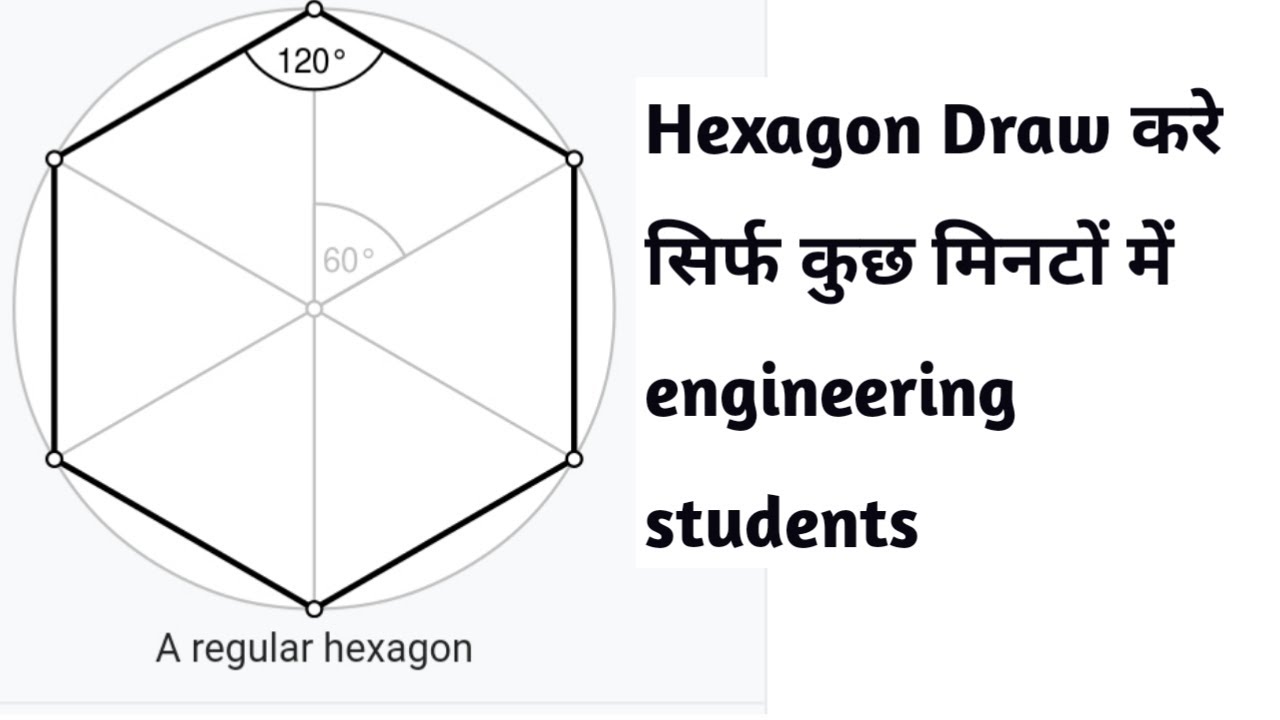
The total sum of its interior angles is 720°, 6 exterior angles, each of 60°, 9 diagonals, and 6 lines of symmetry. A regular hexagon has 6 equal sides, 6 equal interior angles each of 120°.


 0 kommentar(er)
0 kommentar(er)
